Oberseminar Benjamin Bode - Links of non-degenerate polynomials

with R.N. Aranjo dos Santos, E.L Sanches Quiceno

1. Introduction

Definition Complex Singularity

Let $f:{\mathbb{C}}^2\to \mathbb{C}$ be a complex polynomial. We examine the roots of $f$. $f$ has an isolated singularity at the origin $0$ if

1. $f(0)=0$
2. $Jf(0)=0$ (Jacobi-Matrix)
3. There is a nbhd. of $0$ st. $Jf(x)$ has full rank für every $x\in U\backslash \{0\}$ We visualize the boundary of the nbhd. as a small circle $S^3$ around $0$

it seems like singularities are the points where the set $f^{-1}(0)$ looks non-differentiable.

Definition Link of a singularity

We can now define a link in the following way $L_g=f^{-1}(0)\cap S^3$

Example

$f(u,v)=u^p-v^q$ implies a torus link $L_g\cong T_{p,q}$

Statement of this talk: The nondegeneracy of a polynomial implies that higher oder terms do not affect the link. Motivating Question: Complex polynomials are well understood. What if we use real polynomials $f:{\mathbb{R}}^4\to {\mathbb{R}}^2$? In this case most above definitions can be easily corrected. However, reals functions allowmore freedom, allowing the definition of weak singularities.

Definiton Weakly Real Singularity

Let $f:{\mathbb{R}}^4\to {\mathbb{R}}^2$ be a real polynomial. We examine the roots of $f$. $f$ has an isolated singularity at the origin $0$ if

1. $f(0)=0$
2. $Jf(0)=0$ (Jacobi-Matrix)
3. There is a nbhd. of $0$ st. $Jf(x)$ has full rank in $x$ ($⟺x$ regular) for every $x\in (U\backslash \{0\})\cap f^{-1}(0)$

Up until now, the possible singularity links have been classified as follows.

	complex	real weakly isolated sing.	isol. sing.
all polynomials	algebraic links	all	?
non-degen polynomials	unions of torus links	This is the topic of this talk	?

2. Newton boundary

Definition Support of a complex polynomial

Let $f:{\mathbb{C}}^2\to \mathbb{C}$ be a complex polynomial $\sum f(u,v)=c_{m,n}{u}^m{v}^n$. The Support of $f$ ist defined as follows: $\text{supp}(f)=\{(m,n)\in {\mathbb{N}}_0^2:c_{m,n}\ne 0\}$

Definition complex Newton polygon & boundary of $f$

The Newton Hull is defined as: ${\Gamma }_{+}(f):=\text{convex hull of supp}(f)+{\mathbb{R}}_{+}^2$ This results in the Newton Polygon, which is not compact (it goes to infinity on the upper and right side). Therefore we define the Newton Boundary $\Gamma (f)$ as the union of compact $0$ or $1$-faces of the boundary of ${\Gamma }_{+}(f)$. This gets rid of $x,y$-Axes (including segments sittings of those axes).

The Newton boundary becomes a sequence of lines connecting points. It defines an equivalence relation on complex polygons.

The real support and real Newton boundary will be defined analogously

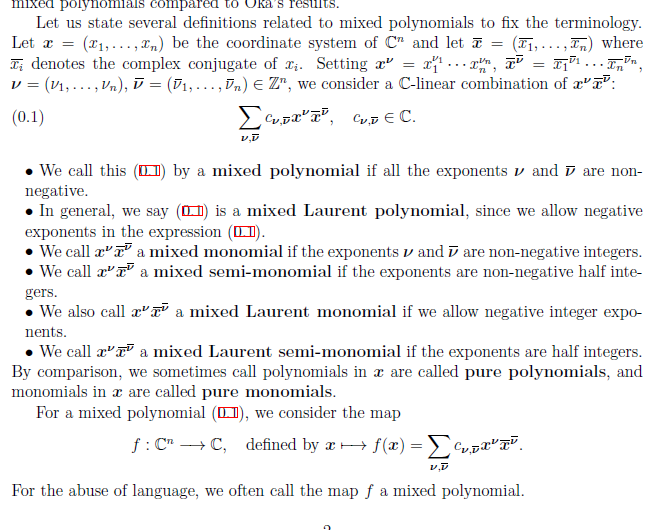
Definition Support of a complex polynomial

Let $f:{\mathbb{R}}^4\to {\mathbb{R}}^2$ be a real polynomial written as “mixed polynomial” $\sum f(u,v)=c_{m_1,m_2,n_1,n_2}{u}^{m_1}{u}^{-m_2}{v}^{n_1}{v}^{-n_2}$. The Support of $f$ ist defined as follows: $\text{supp}(f)=\{(m_1+m_2,n_1+n_2)\in {\mathbb{N}}_0^2:c_{m_1,m_2,n_1,n_2}\ne 0\}$

I dont quite get the way mixed polynomials work. I took the following from Toshizumi, 2023. Anyways the analysis of real functions wont be that important for the following definitions.

For every $0$ or $1$ dimensional face of the Newton boundary $\Gamma (f)$ we can define the face function $f_{\Delta }$ as the sum of monomials of $f$ with support in $\Delta$.

Non-degenerate Functions

Let $f:{\mathbb{C}}^2\to \mathbb{C}$ a mixed polynomial, $\Delta$ a face of $\Gamma (f)$. $f_{\Delta }$ ist nondegenerate if it has no critical points in $(\mathbb{C}\backslash \{0\}{)}^2\cap f_{\Delta }^{-1}(0)$. $f$ is non-degenerate if $f_{\Delta }$ is non-deg for all faces $\Delta$.

Definition

$f:{\mathbb{C}}^2\to \mathbb{C}$ a mixed polynomials. $f$ ist called convenient if it has support on both coordinate axes.

Theorem (Oka)

Let $f$ be mixed, convenient and non-degenerate. Then $f$ has a weakly isolated singularity.

3. Nesting of Links

Deifnition

$f$ mixed, convenient, non-deg. $f$ is called nice if $f_{\Delta }$ has no zeros in $(C-\{0\}{)}^2$ for all $0$-faces that do not lie on coordinate axes.

radially weighted homogenous

$f$ ist radially weighted homogenous iff. there is a $p_1,p_2\in \mathbb{N},gcd(p_1,p_2)=1,d\in \mathbb{N}$ s.t. $f({\lambda }^{p_1}u,{\lambda }^{p_2}v)={\lambda }^d(u,v)$.

This is true iff $supp(f)$ lies on a straight (non horizontal and non vertical) line

We notice that every $1$-dimensional segment of the Newton boundary has a unique vector $p=(p_1,p_2)$ orthogonal and pointing to the inside of the Newton polygon.

Theorem (authors)

$f$ a complex mixed, convenient, nice, non-deg polym as always with $N$ $1$-faces (with boundaries ${\Lambda }_1,{\Lambda }_2$) in $\Gamma (f)$. Let

• $X_1=\{(u,v\in S^3:|u{|}^2<\frac{1}{N}\}\cong S^1\times D$
• $X_i:=\{(u,v)\in S^3:\frac{i-1}{N}<<|u{|}^2<\frac{i}{N}\}\cong [0,1[\times T^2$
• $X_N:=\{(u,v)\in S^3:\frac{N-1}{N}<<|u{|}^2\le 1\}\cong S^1\times D$

They fulfil $S^3={⨆}_{i=1}^N{X}_i$ We define the following sequence of links

• $L_1:=f_{{\Lambda }_1}^{-1}(0)\cap S^3-\{0\}\cong X_1$
• $L_i:=f_{{\Lambda }_i}^{-1}(0)\cap S^3-\{u=0\wedge v=0\}\cong X_i$
• $L_1:=f_{{\Lambda }_N}^{-1}(0)\cap S^3-\{u=0\}\cong X_n$

Then the link of the singularity of $f$ is $L=L_1\cup L_2\cup ...\cup L_N$ where $L_i\subset X_i$.

4. Classification

Definition

Let ${\tau }_1(u,v)=(-u,v),{\tau }_2(u,v)=(u,-v),{\tau }_3(u,v)=(-u,-v)$. Let $M\subseteq {\mathbb{C}}^2$ be a submanifold setwise invariant under ${\tau }_1,{\tau }_2,{\tau }_3$.

• A link in $M$ is called even if it is setwise invariant under ${\tau }_1$ or ${\tau }_2$
• A link in $M$ is called odd if it is setwise invariant under or ${\tau }_3$
• A link in $M$ is called fixed-point-free if it does not contain any fixed points of ${\tau }_i$.

Theorem (author)

A link $L$ in $S^3$ is the links of a weak i.sing. of some $f$ mixed, conv, non-deg, nice iff (not sure if this is an and or or)

• $L$ is fixed-point-free even
• $L$ is odd
• $L$ is as in the previous them with every $L_i$ fixed-pt-free even or odd.